The Physics Teacher
November 1993
Volume 31/Number 8

The Physics of Bungee Jumping

Paul G. Menz

The vocabulary is new and multisyllabic - slingshotting, sandbagging, bodydipping, vinejumping, part-of-four, and more. The spelling is erratic - bungee, bungi, bungy. And terra firma types would say the trendy activity is a threat to body wholeness. But physicists, of course, calmly regard bungee jumping as a dramatic demonstration of the conversation of energy. They know that gravitational potential energy at the top of the jump is converted to elastic potential energy at the bottom. The basic equations involved have been used for years to describe events in which loads are suddenly applied to springs. The bungee cord is simply a very weak spring yielding large spring deflections and rather small force magnitudes. But for the benefit of those who didn't know this, we'll lay it all out here.

History

The origin of sport bungee jumping is quite recent, but the activity is related to the centuries-old ritualistic practices of "land divers" of Pentecost Island in the Pacific Archipelago of Vanuatu. There the men demonstrate their courage and offer their injuries to the gods for a plentiful harvest of yams. But it was members of the Oxford University Dangerous Sport Club who, inspired by a film about "vine jumpers," plummeted off a bridge near Bristol, England, in April 1979 and thereby launched a new worldwide recreational activity. During the 1980s, the sport flourished in New Zealand and France and was brought into the United States by John and Peter Kockelman of California. In the early 1990s facilities sprang up all over this country with cranes, towers, and hot-air balloons serving as platforms. Thousands have now experienced that "ultimate adrenaline rush." Many have tried to describe that exhilaration. All share the post-jump elation and grin. In Fig. 1 the jumper, following countdown, has leapt backwards. In Fig. 2, the jumper is enjoying that motionless instant at the top of the rebound.

Equipment

Bungee cords have some vague military origin, but today can be purchased from manufacturers who construct them specifically for jumping. They are soft and springy and may stretch to three or four times their free length. The harnesses are related to and derive from mountain climbing equipment, as does the carabiner, which is the principal link between the cord and the harness. Most present-day facilities use redundant connections, that is, double hookups to the jumper's body are provided as shown in Figs. 3 and 4. If a jumper chooses an ankle jump, the body harness is backup; if the body harness is primary, the chest/shoulder harness is secondary.

Safety

This article is not a commercial for bungee jumping, but familiarity with the equipment used and the forces involved may surprise some readers and may testify to the safety of this activity as a sport. The activity was banned in France after three deaths in 1989. The Australian government declared a hiatus after an accident in 1990, and the summer of 1992 saw a few accidents in the United States that were given major exposure by the media and caused several state governments to get involved. But the activity is clearly basically safe. All accidents can be traced to human error as related to improper attachment, mismatch between jumper and cord, total height of jump available, misunderstanding or miscalculation of the physics involved, and other lapses. This view is shared by Carl Finocchiaro, a registered professional engineer who operates Sky Tower Engineering Inc. and has been professionally involved in this sport for several years. He is a charter member of the North American Bungee Association and is the original and incumbent chairman of its safety committee. He has stated, "I have investigated many accidents and can confidently conclude that all are caused by human error and not faulty equipment."

Minor injuries such as skin burn, which is caused by gripping the cord, occur when jumpers act contrary to instructions. Some jumpers reported getting slapped in the face by the cord. But serious injury inflicted by the cord, such as strangulation, appears not to happen. This can be explained by a combination of factors, including:

1. the cord's minimal torsional stiffness
2. some pendulum motion, which tends to keep the cord away from the jumper
3. the fact that any entanglement will occur when the cord is slack, and will be gradually and gently unwrapped and forgiven as the cord develops elongation and associated low tensile force.

No modern-day jump site has seen any serious entanglement, and it is noteworthy that many participants enjoy somersaulting during the free fall without any detrimental effects.

Some daredevilish embellishments may tempt the adventurous participants. "Slingshotting" (from the ground up), "sandbagging" (jumping with extra weight), and "bodydipping" (over water) are examples. Extreme care and proper application of the physics involved are vitally important in these challenges.

Physics of Bungee Jumping

The principle components in the physics of this sport are the gravitational potential energy of the jumper and the elastic potential of the stretched cord.

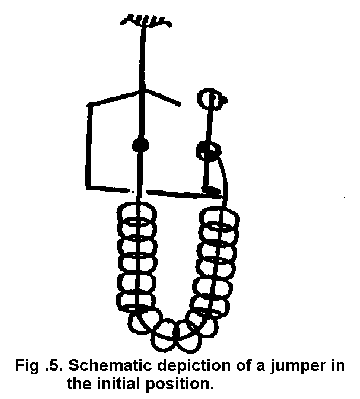
Figure 5 depicts a jumper of mass m who is tethered by a bungee cord conveniently attached to the supporting structure on a level with her center of mass.

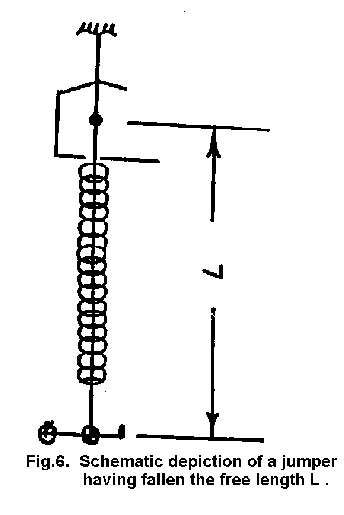
Figure 6 depicts the jumper just as she has fallen a distance equal to the free length (L) of the cord. This event terminates the free fall, which lasts between one and two seconds.

Figure 7 depicts the jumper at the bottom extremity of the jump. The jumper has fallen a total distance of L + d, the cord has stretched a distance d, and the velocity of everything at that instant is equal to zero.

Energy considerations dictate that the gravitational potential energy of the jumper in the initial state is equal to the elastic potential of the cord in the final state. Therefore:

If we allow the bungee cord to be a linear spring of stiffness K N/m, then

and from this the following quadratic equation is produced:

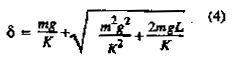
When a given cord (K,L) is matched with a given person (m), then the d will be determined by

When a given jump height (L + d) is to be matched with a given person (m), then the stiffness (K) will be determined by

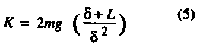
In many cases, the first match is made so that the total fall (L + d) will fit the facility, but in order to show the orders of magnitude involved, consider a hypothetical second match between a person (m) and a jump height (L + d). Suppose a person weighing 667 N is to jump using a 9-m cord which will stretch 18 m and use a jump height of 27 m:

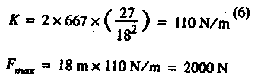
This 200% elongation produces a maximum force three times the jumper's weight. A 300% elongation is a softer ride:

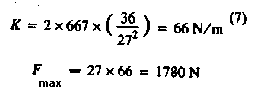
This requires a jump height of 36 m, and a maximum acceleration of 2.7 g's is produced.

Calculations Closer to Reality

In a more realistic vein, two factors must be considered:

1. A given facility will have a limited number of cords of differing lengths and stiffnesses.
2. Those cords have been found to demonstrate variable stiffness over their range of use.

Some actual force-deflection diagrams are shown in Fig. 8,

and a piece wise linear approximation is shown in Fig. 9. Three areas are delineated in Fig. 9 so that Fdx can be evaluated as a sum of areas:

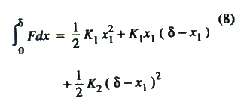
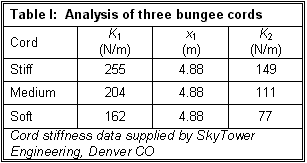
Analysis of these three bungee cords yields the information found in Table I.

A third factor, which complicates the arithmetic but contributes in two ways to a facility's improvement, is the addition of a static line (see Fig. 10).

This rigid line or cable prevents the cord itself from rubbing or chafing against the floor of the basket or tower. It also may be played in or out to customize the jump height to any individual. The conservation of energy then takes this form:

which yields the following quadratic equation:

It seems plausible to match a heavy person (1112 N) with the stiff cord, a medium person (800 N) with the medium cord, and the soft cord with a jumper of about 490 N. The calculations have been performed using a free length (L) of 9 m, a static cable length (Ls) of 1.8 m, and the matched jumpers and cords. The results are presented in Table II, which shows the maximum forces and the number of g's obtainable from Eqs. (9) and (10) respectively

Some organizations might give the light jumper a 27- to 28-m ride to match the geometry of a given tower or crane. This can be done by adding length to the static line. Suppose Ls is increased to 3.6 m for the 490-N jumper. Then d = 14.5 m, the jump height increases to 26.8 m, and the g value becomes 3.13.

Discussion

We see from both the hypothetical linear spring and the real nonlinear spring that the proper match of cord and jumper should produce maximum accelerations of the order of 3 g's, and with a cord of about 9 m free length, the jump height should be approximately 28 m.

The material presented here offers the instructor of introductory physics an "in-vogue" application of the conservation of energy concept that is related to both entertainment and athleticism. Also, perhaps a teacher could devise an appealing laboratory exercise using the same applications for short bungee cords (0.6 m or so) and masses from the stockroom (with due attention being given to the masses as they rebound!).

Finally, it is informative to consider Eq. (4) carefully because it depicts one of the most important practical ideas to be learned from dynamics, whether or not it is related to bungee jumping. Equation (4) is applicable to any weight mg being dropped onto a compression spring of stiffness K from distance L above. Even if dropped from a distance of L=0, the mass creates a d of 2 mg/K; which is exactly twice the effect realized if the mass is applied gradually. In other words, if a load is administered to any system or structure suddenly or dynamically, the force imposed is, at the minimum, twice as great as the static load.

References

1. David Thigpen, Time, April 23, 1990, p. 75.
2. Jeremy Hart, World, May, 1991, pp. 40-45.
3. Carl Finocchiaro, Sky Tower Engineering, Inc., 1340 Dahlia Street, Denver, CO 80220, "Engineering Report," March 3, 1992.
4. 4. F.W. Sears and M.W. Zemansky, University Physics, 3rd ed. (Addison-Wesley, Reading, MA, 1963), p. 174.